maths number problem solving

• Solutions Integral Calculator Derivative Calculator Algebra Calculator Matrix Calculator More...
• Graphing Line Graph Exponential Graph Quadratic Graph Sine Graph More...
• Calculators BMI Calculator Compound Interest Calculator Percentage Calculator Acceleration Calculator More...
• Geometry Pythagorean Theorem Calculator Circle Area Calculator Isosceles Triangle Calculator Triangles Calculator More...
• Tools Notebook Groups Cheat Sheets Worksheets Study Guides Practice Verify Solution

• Pre Algebra Order of Operations Factors & Primes Fractions Long Arithmetic Decimals Exponents & Radicals Ratios & Proportions Percent Modulo Number Line Expanded Form Mean, Median & Mode
• Algebra Equations Inequalities System of Equations System of Inequalities Testing Solutions Basic Operations Algebraic Properties Partial Fractions Polynomials Rational Expressions Sequences Power Sums Interval Notation Pi (Product) Notation Induction Prove That Logical Sets Word Problems
• Pre Calculus Equations Inequalities Scientific Calculator Scientific Notation Arithmetics Complex Numbers Coterminal Angle Polar/Cartesian Simultaneous Equations System of Inequalities Polynomials Rationales Functions Arithmetic & Comp. Coordinate Geometry Plane Geometry Solid Geometry Conic Sections Trigonometry
• Calculus Derivatives Derivative Applications Limits Integrals Integral Applications Integral Approximation Series ODE Multivariable Calculus Laplace Transform Taylor/Maclaurin Series Fourier Series Fourier Transform
• Functions Line Equations Functions Arithmetic & Comp. Conic Sections Transformation
• Linear Algebra Matrices Vectors
• Trigonometry Quadrant Identities Proving Identities Trig Equations Trig Inequalities Evaluate Functions Simplify
• Statistics Mean Geometric Mean Quadratic Mean Average Median Mode Order Minimum Maximum Probability Mid-Range Range Standard Deviation Variance Lower Quartile Upper Quartile Interquartile Range Midhinge Standard Normal Distribution
• Physics Mechanics
• Chemistry Chemical Reactions Chemical Properties
• Finance Simple Interest Compound Interest Present Value Future Value
• Economics Point of Diminishing Return
• Conversions Roman Numerals Radical to Exponent Exponent to Radical To Fraction To Decimal To Mixed Number To Improper Fraction Radians to Degrees Degrees to Radians Hexadecimal Scientific Notation Distance Weight Time Volume
• Pre Algebra
• Pre Calculus
• Linear Algebra
• Trigonometry
• Conversions

Number Line

• x^{2}-x-6=0
• -x+3\gt 2x+1
• line\:(1,\:2),\:(3,\:1)
• prove\:\tan^2(x)-\sin^2(x)=\tan^2(x)\sin^2(x)
• \frac{d}{dx}(\frac{3x+9}{2-x})
• (\sin^2(\theta))'
• \lim _{x\to 0}(x\ln (x))
• \int e^x\cos (x)dx
• \int_{0}^{\pi}\sin(x)dx
• \sum_{n=0}^{\infty}\frac{3}{2^n}
• Is there a step by step calculator for math?
• Symbolab is the best step by step calculator for a wide range of math problems, from basic arithmetic to advanced calculus and linear algebra. It shows you the solution, graph, detailed steps and explanations for each problem.
• Is there a step by step calculator for physics?
• Symbolab is the best step by step calculator for a wide range of physics problems, including mechanics, electricity and magnetism, and thermodynamics. It shows you the steps and explanations for each problem, so you can learn as you go.
• How to solve math problems step-by-step?
• To solve math problems step-by-step start by reading the problem carefully and understand what you are being asked to find. Next, identify the relevant information, define the variables, and plan a strategy for solving the problem.
• My Notebook, the Symbolab way Math notebooks have been around for hundreds of years. You write down problems, solutions and notes to go back...

We want your feedback

Please add a message.

Message received. Thanks for the feedback.

• Solve equations and inequalities
• Simplify expressions
• Factor polynomials
• Graph equations and inequalities
• Advanced solvers
• All solvers
• Arithmetics
• Determinant
• Percentages
• Scientific Notation
• Inequalities

What can QuickMath do?

QuickMath will automatically answer the most common problems in algebra, equations and calculus faced by high-school and college students.

• The algebra section allows you to expand, factor or simplify virtually any expression you choose. It also has commands for splitting fractions into partial fractions, combining several fractions into one and cancelling common factors within a fraction.
• The equations section lets you solve an equation or system of equations. You can usually find the exact answer or, if necessary, a numerical answer to almost any accuracy you require.
• The inequalities section lets you solve an inequality or a system of inequalities for a single variable. You can also plot inequalities in two variables.
• The calculus section will carry out differentiation as well as definite and indefinite integration.
• The matrices section contains commands for the arithmetic manipulation of matrices.
• The graphs section contains commands for plotting equations and inequalities.
• The numbers section has a percentages command for explaining the most common types of percentage problems and a section for dealing with scientific notation.

Math Topics

More solvers.

• Add Fractions
• Simplify Fractions

• HW Guidelines
• Study Skills Quiz
• Find Local Tutors
• Demo MathHelp.com
• Join MathHelp.com

Select a Course Below

• ACCUPLACER Math
• Math Placement Test
• PRAXIS Math
• + more tests
• 5th Grade Math
• 6th Grade Math
• Pre-Algebra
• College Pre-Algebra
• Introductory Algebra
• Intermediate Algebra
• College Algebra

"Number" Word Problems

What are "number" word problems.

Number word problems involve relationships between different numbers; these exercises ask you to find some number (or numbers) based on those relationships.

Content Continues Below

MathHelp.com

Algebra Word Problems

How do you solve number word problems?

To set up and solve number word problems, it is important clearly to label variables and expressions, using your translation skills to convert the words into algebra. The process of clear labelling will often end up doing nearly all of the work for you.

Number word problems are usually fairly contrived, but they're also fairly standard. Keep in mind that the point of these exercises isn't their relation to "real life", but rather the growth of your ability to extract the mathematics from the English. These exercises are a great way to stretch your mental muscles, use what you know already, apply your logic (and common sense), and then hippity-hop your way to the answer.

What is an example of solving a number word problem?

• The sum of two consecutive integers is 15 . Find the numbers.

They've given me many pieces of information here.

• I'm adding (that is, summing) two things
• the numbers are integers (like −3 and 6 )
• the second number is 1 more than the first
• the result of the addition will be 15

Advertisement

How do I know that the second number will be larger than the first by 1 ? Because the two integers are "consecutive", which means "one right after the other, not skipping over anything between". (Examples of consecutive integers would be −12 and −11 , 1 and 2 , and 99 and 100 .)

The "integers" are the number zero, the whole numbers, and the negatives of the whole numbers. In going from one integer to the next consecutive integer, I'll have gone up by one unit.

I need to figure out what are the two numbers that I'm adding. The second number is defined in terms of the first number, so I'll pick a variable to stand for this number that I don't yet know:

1st number: n

The second number is one more than the first, so my expression for the second number is:

2nd number: n + 1

I know that I'm supposed to add these two numbers, and that the result will be (in other words, I should set the sum equal to) 15 . This, along with my translation skills, allows me to create an equation, being the algebraic equivalent to "(this number) added to (the next number) is (fifteen)":

n + ( n + 1) = 15

This is a linear equation that I can solve :

2 n + 1 = 15

The exercise did not ask me for the value of the variable n ; it asked for the identity of two numbers. So my answer is not " n  = 7 "; the actual answer, taking into account the second number, too, is:

The numbers are 7 and 8 .

It usually isn't required that you write your answer out like this; sometimes a very minimal " 7, 8 " is regarded as acceptible form. But the exercise asked me, in complete sentences, a question about two numbers; I feel like it's good form to answer that question in the form of a complete sentence.

What do they mean when they say "consecutive even (or odd) integers"?

Some number word problems will refer to "consecutive even (or odd) integers". This means that they're talking about two whole numbers (or their negatives) that are both even or else both odd; in particular, the two numbers are 2 units apart.

• The product of two consecutive negative even integers is 24 . Find the numbers.

I'll start with extracting the information they've given me.

• I'm multiplying (that is, finding the product of) two things
• those two things are numbers
• those two numbers are integers
• those two integers are even
• those two even integers are negative
• the second even integer is 2 units more than the first
• when I multiply, I'll get 24

How do I know that one number will be 2 more than the other? Because these numbers are consecutive even integers; the "consecutive" part means "the one right after the other", and the "even" part means that the numbers are two units apart. (Examples of consecutive even integers are 10 and 12 , −14 and −16 , and 0 and 2 .)

The second number is defined in terms of the first number, so I'll pick a variable for the first number. Then the second number will be two units more than this.

1st number: n 2nd number: n + 2

When I multiply these two numbers, I'm supposed to get 24 . This gives me my equation:

( n )( n + 2) = 24

This is a quadratic equation that I can solve :

( n )( n + 2) = 24 n 2 + 2 n = 24 n 2 + 2 n − 24 = 0 ( n + 6)( n − 4) = 0

This equation clearly has two solutions, being n  = −6 and n  = 4 . Since the numbers I am looking for are negative, I can ignore the " 4 " solution value and instead use the n  = −6 solution.

Then the next number, being larger than the first number by 2 , must be n  + 2 = −4 , and my answer is:

The numbers are −6 and −4 .

In the exercise above, one of the solutions to the exercise — namely, n  = −6 — was one of the solutions to the equation; the other solution to the equation — namely, n  = 4 — had the sign opposite to the other answer to the exercise.

You will encounter this pattern often in solving this type of word problem. However, do not assume that you can use both solutions if you just change the signs to be whatever you think they ought to be. While this often works, it does not always work, and it's sure to annoy your grader. Instead, throw out invalid results, and solve properly for the valid ones.

• Twice the larger of two numbers is three more than five times the smaller, and the sum of four times the larger and three times the smaller is 71 . What are the numbers?

The point of exercises like this is to give me practice in unwrapping and unwinding these words, somehow turning the words into algebraic expressions and equations. The point is in the setting-up and solving, not in the relative "reality" of the exercise. That said, how do I solve this? The best first step is to start labelling.

I need to find two numbers and, this time, they haven't given me any relationship between the two, like "two consecutive even integers". Since neither number is defined by the other, I'll need two letters to stand for the two unknowns. I'll need to remember to label the variables with their definitions.

the larger number:  x

the smaller number:  y

Now I can create expressions and then an equation for the first relationship they give me:

twice the larger:  2 x

three more than five times the smaller:  5 y + 3

relationship between ("is"):  2 x = 5 y + 3

And now for the other relationship they gave me:

four times the larger:  4 x

three times the smaller:  3 y

relationship between ("sum of"):  4 x + 3 y = 71

Now I have two equations in two variables:

2 x = 5 y + 3

4 x + 3 y = 71

I will solve, say, the first equation for x = :

x = (5/2) y + (3/2)

(There's no right or wrong in this choice; it's just what I happened to choose while I was writing up this page.)

Then I'll plug the right-hand side of this into the second equation in place of the x :

4[ (5/2) y + (3/2) ] + 3 y = 71

10 y + 6 + 3 y = 71

13 y + 6 = 71

y = 65/13 = 5

Now that I have the value for y , I can back-solve for x :

x = (5/2)(5) + (3/2)

x = (25/2) + (3/2)

x = 28/2 = 14

As always, I need to remember to answer the question that was actually asked. The solution here is not " x  = 14 ", but is instead the following:

larger number: 14

smaller number: 5

What are the steps for solving "number" word problems?

The steps for solving "number" word problems are these:

• Read the exercise through once; don't try to start solving it before you even know what it says.
• Figure out what you know (for instance, are you adding or multiplying?).
• Figure out what you don't know; this will probably be the value(s) of number(s).
• Pick one or more useful variables for unknown(s) that you need to find.
• Use the variable(s) and the known information to create expressions.
• Use these expressions and the known information to create one or more equations.
• Solve the equation(s) for the unknown(s).
• Check your definition(s) for your variable(s).
• Use this/these definition(s) to state your answer in clear terms.

But more than any list, the trick to doing this type of problem is to label everything very explicitly. Until you become used to doing these, do not attempt to keep track of things in your head. Do as I did in this last example: clearly label every single step; make your meaning clear not only to the grader but to yourself. When you do this, these problems generally work out rather easily.

URL: https://www.purplemath.com/modules/numbprob.htm

Standardized Test Prep

College math, homeschool math, share this page.

• Terms of Use
• Privacy / Cookies
• About Purplemath
• About the Author
• Tutoring from PM
• Advertising
• Linking to PM
• Site licencing

Visit Our Profiles

High Impact Tutoring Built By Math Experts

Personalized standards-aligned one-on-one math tutoring for schools and districts

Free ready-to-use math resources

Hundreds of free math resources created by experienced math teachers to save time, build engagement and accelerate growth

20 Effective Math Strategies To Approach Problem-Solving 

Katie Keeton

Math strategies for problem-solving help students use a range of approaches to solve many different types of problems. It involves identifying the problem and carrying out a plan of action to find the answer to mathematical problems.  

Problem-solving skills are essential to math in the general classroom and real-life. They require logical reasoning and critical thinking skills. Students must be equipped with strategies to help them find solutions to problems.

This article explores mathematical problem solving strategies, logical reasoning and critical thinking skills to help learners with solving math word problems independently in real-life situations. 

What are problem-solving strategies?

Problem-solving strategies in math are methods students can use to figure out solutions to math problems. Some problem-solving strategies: 

• Draw a model
• Use different approaches
• Check the inverse to make sure the answer is correct

Students need to have a toolkit of math problem-solving strategies at their disposal to provide different ways to approach math problems. This makes it easier to find solutions and understand math better. 

Strategies can help guide students to the solution when it is difficult ot know when to start.

The ultimate guide to problem solving techniques

Download these ready-to-go problem solving techniques that every student should know. Includes printable tasks for students including challenges, short explanations for teachers with questioning prompts.

20 Math Strategies For Problem-Solving

Different problem-solving math strategies are required for different parts of the problem. It is unlikely that students will use the same strategy to understand and solve the problem. 

Here are 20 strategies to help students develop their problem-solving skills. 

Strategies to understand the problem

Strategies that help students understand the problem before solving it helps ensure they understand: 

• The context
• What the key information is
• How to form a plan to solve it

Following these steps leads students to the correct solution and makes the math word problem easier .

Here are five strategies to help students understand the content of the problem and identify key information. 

1. Read the problem aloud

Read a word problem aloud to help understand it. Hearing the words engages auditory processing. This can make it easier to process and comprehend the context of the situation.

2. Highlight keywords 

When keywords are highlighted in a word problem, it helps the student focus on the essential information needed to solve it. Some important keywords help determine which operation is needed.  For example, if the word problem asks how many are left, the problem likely requires subtraction.  Ensure students highlight the keywords carefully and do not highlight every number or keyword. There is likely irrelevant information in the word problem.

3. Summarize the information

Read the problem aloud, highlight the key information and then summarize the information. Students can do this in their heads or write down a quick summary.  Summaries should include only the important information and be in simple terms that help contextualize the problem.

4. Determine the unknown

A common problem that students have when solving a word problem is misunderstanding what they are solving. Determine what the unknown information is before finding the answer.  Often, a word problem contains a question where you can find the unknown information you need to solve. For example, in the question ‘How many apples are left?’ students need to find the number of apples left over.

5. Make a plan

Once students understand the context of the word problem, have dentified the important information and determined the unknown, they can make a plan to solve it.  The plan will depend on the type of problem. Some problems involve more than one step to solve them as some require more than one answer.  Encourage students to make a list of each step they need to take to solve the problem before getting started.

Strategies for solving the problem 

1. draw a model or diagram.

Students may find it useful to draw a model, picture, diagram, or other visual aid to help with the problem solving process.  It can help to visualize the problem to understand the relationships between the numbers in the problem. In turn, this helps students see the solution.

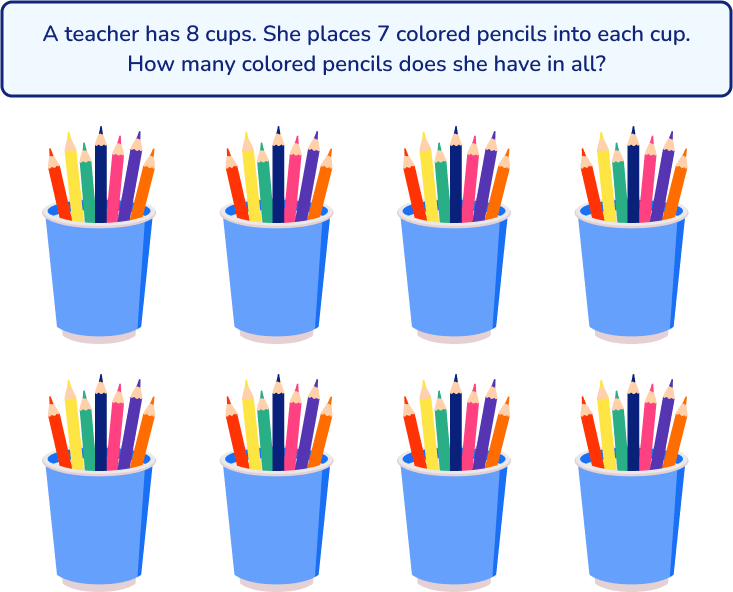
Similarly, you could draw a model to represent the objects in the problem:

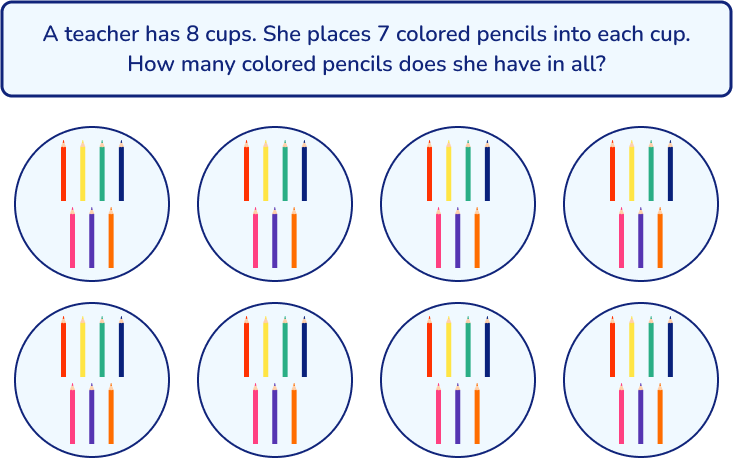
2. Act it out

This particular strategy is applicable at any grade level but is especially helpful in math investigation in elementary school . It involves a physical demonstration or students acting out the problem using movements, concrete resources and math manipulatives .  When students act out a problem, they can visualize and contectualize the word problem in another way and secure an understanding of the math concepts.  The examples below show how 1st-grade students could “act out” an addition and subtraction problem:

3. Work backwards

Working backwards is a popular problem-solving strategy. It involves starting with a possible solution and deciding what steps to take to arrive at that solution.  This strategy can be particularly helpful when students solve math word problems involving multiple steps. They can start at the end and think carefully about each step taken as opposed to jumping to the end of the problem and missing steps in between.

For example,

To solve this problem working backwards, start with the final condition, which is Sam’s grandmother’s age (71) and work backwards to find Sam’s age. Subtract 20 from the grandmother’s age, which is 71.  Then, divide the result by 3 to get Sam’s age. 71 – 20 = 51 51 ÷ 3 = 17 Sam is 17 years old.

4. Write a number sentence

When faced with a word problem, encourage students to write a number sentence based on the information. This helps translate the information in the word problem into a math equation or expression, which is more easily solved.  It is important to fully understand the context of the word problem and what students need to solve before writing an equation to represent it.

5. Use a formula

Specific formulas help solve many math problems. For example, if a problem asks students to find the area of a rug, they would use the area formula (area = length × width) to solve.   Make sure students know the important mathematical formulas they will need in tests and real-life. It can help to display these around the classroom or, for those who need more support, on students’ desks.

Strategies for checking the solution 

Once the problem is solved using an appropriate strategy, it is equally important to check the solution to ensure it is correct and makes sense. 

There are many strategies to check the solution. The strategy for a specific problem is dependent on the problem type and math content involved.

Here are five strategies to help students check their solutions. 

1. Use the Inverse Operation

For simpler problems, a quick and easy problem solving strategy is to use the inverse operation. For example, if the operation to solve a word problem is 56 ÷ 8 = 7 students can check the answer is correct by multiplying 8 × 7. As good practice, encourage students to use the inverse operation routinely to check their work. 

2. Estimate to check for reasonableness

Once students reach an answer, they can use estimation or rounding to see if the answer is reasonable.  Round each number in the equation to a number that’s close and easy to work with, usually a multiple of ten.  For example, if the question was 216 ÷ 18 and the quotient was 12, students might round 216 to 200 and round 18 to 20. Then use mental math to solve 200 ÷ 20, which is 10.  When the estimate is clear the two numbers are close. This means your answer is reasonable. 

3. Plug-In Method

This method is particularly useful for algebraic equations. Specifically when working with variables.  To use the plug-in method, students solve the problem as asked and arrive at an answer. They can then plug the answer into the original equation to see if it works. If it does, the answer is correct.

If students use the equation 20m+80=300 to solve this problem and find that m = 11, they can plug that value back into the equation to see if it is correct. 20m + 80 = 300 20 (11) + 80 = 300 220 + 80 = 300 300 = 300 ✓

4. Peer Review

Peer review is a great tool to use at any grade level as it promotes critical thinking and collaboration between students. The reviewers can look at the problem from a different view as they check to see if the problem was solved correctly.   Problem solvers receive immediate feedback and the opportunity to discuss their thinking with their peers. This strategy is effective with mixed-ability partners or similar-ability partners. In mixed-ability groups, the partner with stronger skills provides guidance and support to the partner with weaker skills, while reinforcing their own understanding of the content and communication skills.  If partners have comparable ability levels and problem-solving skills, they may find that they approach problems differently or have unique insights to offer each other about the problem-solving process.

5. Use a Calculator

A calculator can be introduced at any grade level but may be best for older students who already have a foundational understanding of basic math operations. Provide students with a calculator to allow them to check their solutions independently, accurately, and quickly. Since calculators are so readily available on smartphones and tablets, they allow students to develop practical skills that apply to real-world situations.  

Step-by-step problem-solving processes for your classroom

In his book, How to Solve It , published in 1945, mathematician George Polya introduced a 4-step process to solve problems. 

Polya’s 4 steps include:

• Understand the problem
• Devise a plan
• Carry out the plan

Today, in the style of George Polya, many problem-solving strategies use various acronyms and steps to help students recall. 

Many teachers create posters and anchor charts of their chosen process to display in their classrooms. They can be implemented in any elementary, middle school or high school classroom. 

Here are 5 problem-solving strategies to introduce to students and use in the classroom.

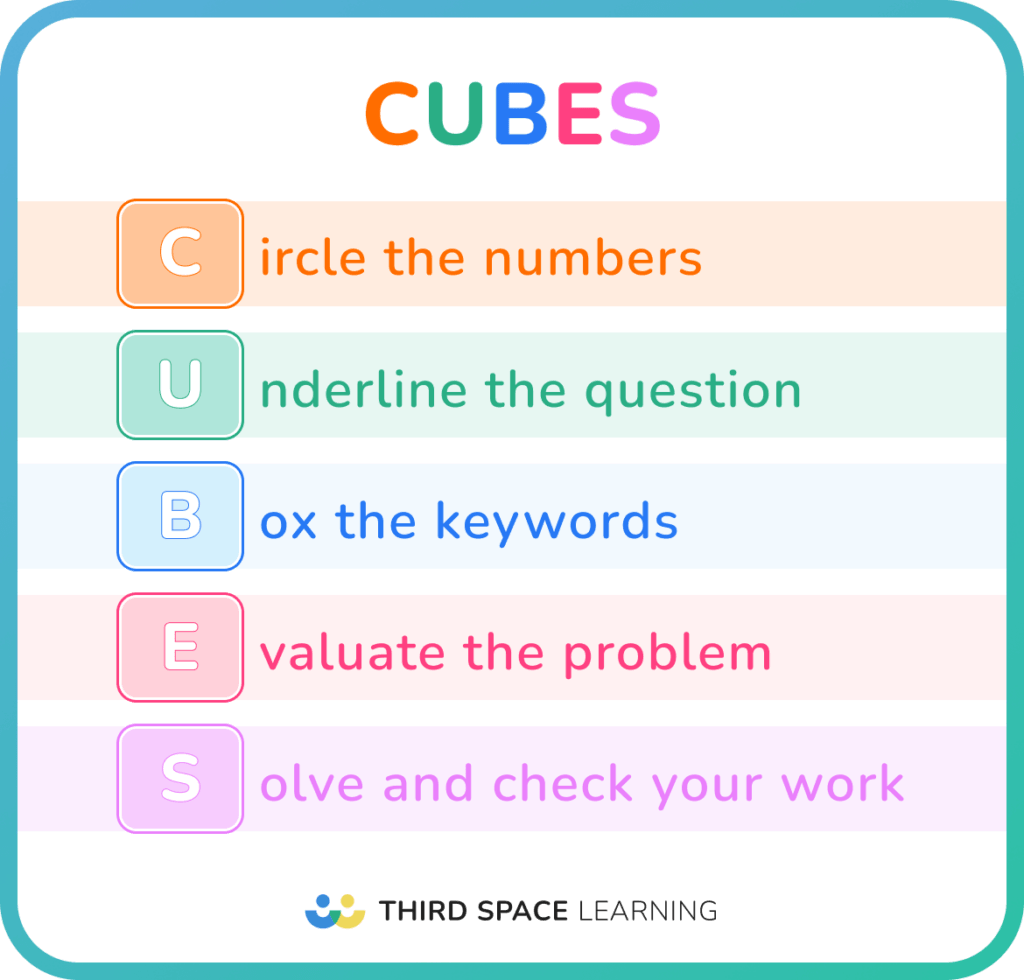
How Third Space Learning improves problem-solving 

Resources .

Third Space Learning offers a free resource library is filled with hundreds of high-quality resources. A team of experienced math experts carefully created each resource to develop students mental arithmetic, problem solving and critical thinking. 

Explore the range of problem solving resources for 2nd to 8th grade students. 

One-on-one tutoring 

Third Space Learning offers one-on-one math tutoring to help students improve their math skills. Highly qualified tutors deliver high-quality lessons aligned to state standards. 

Former teachers and math experts write all of Third Space Learning’s tutoring lessons. Expertly designed lessons follow a “my turn, follow me, your turn” pedagogy to help students move from guided instruction and problem-solving to independent practice. 

Throughout each lesson, tutors ask higher-level thinking questions to promote critical thinking and ensure students are developing a deep understanding of the content and problem-solving skills.

Problem-solving

Educators can use many different strategies to teach problem-solving and help students develop and carry out a plan when solving math problems. Incorporate these math strategies into any math program and use them with a variety of math concepts, from whole numbers and fractions to algebra. 

Teaching students how to choose and implement problem-solving strategies helps them develop mathematical reasoning skills and critical thinking they can apply to real-life problem-solving.

READ MORE :

• 8 Common Core math examples
• Tier 3 Interventions: A School Leaders Guide
• Tier 2 Interventions: A School Leaders Guide
• Tier 1 Interventions: A School Leaders Guide

There are many different strategies for problem-solving; Here are 5 problem-solving strategies: • draw a model  • act it out  • work backwards  • write a number sentence • use a formula

Here are 10 strategies for problem-solving: • Read the problem aloud • Highlight keywords • Summarize the information • Determine the unknown • Make a plan • Draw a model  • Act it out  • Work backwards  • Write a number sentence • Use a formula

1. Understand the problem 2. Devise a plan 3. Carry out the plan 4. Look back

Some strategies you can use to solve challenging math problems are: breaking the problem into smaller parts, using diagrams or models, applying logical reasoning, and trying different approaches.

Related articles

Differentiation Strategies In The Classroom: 8 Methods For Every Teacher

Retrieval Practice: A Foolproof Method To Improve Student Retention and Recall

5 Tried And Tested Strategies To Increase Student Engagement At School

Rote Memorization: Is It Effective In Education?

Ultimate Guide to Metacognition [FREE]

Looking for a summary on metacognition in relation to math teaching and learning?

Check out this guide featuring practical examples, tips and strategies to successfully embed metacognition across your school to accelerate math growth.

Privacy Overview

Please ensure that your password is at least 8 characters and contains each of the following:

• a special character: @$#!%*?&