problem solving maths targets

Math IEP Goals For Special Education

Drafting IEP goals can be difficult, so here are a few math IEP goals (across various ability levels) to get you started. Please adapt and modify to meet the specific needs of your students. Keep in mind a goal should be a skill you believe is achievable by the student in 1 school year. You can always do an addendum if a student has met all criteria for the goal/objectives.

Remember, when writing objectives, break down the goal into smaller steps. You can lessen the percentage of accuracy, the number of trials (3/5 vs 4/5), or amount of prompting. Just make sure the objectives build on each other and are working towards mastery.

The reason why I always list accuracy at 100% when writing Math goals is because the answer is either right or wrong, an answer to a math problem can’t be 50% correct. So feel free to play with the ## of trials for accuracy.

Number Identification:

Goal: Student will independently identify numbers 1-20 (verbally, written, or pointing) with 100% accuracy on 4 out of 5 trials measured quarterly.

Goal: When verbally prompted by teacher to “point to the number _________”, Student will independently select the correct number with 100% accuracy on 4 out of 5 trials measured quarterly.

Goal: Student will independently count in rote order numbers 1-25 with 100% accuracy on 4 out of 5 trials measured quarterly.

Goal: Student will independently count by 2, 3, 5, 10 starting from 0-30 verbally or written, with 100% accuracy on 4 out of 5 trials measured quarterly.

One-to-one Correspondence:

Goal: When given up to 10 objects, Student will independently count and determine how many objects there are (verbally, written, or by pointing to a number) with 100% accuracy on 4 out of 5 trials measured quarterly/monthly.

Goal: When given up to 10 items/objects, Student will independently count and move the items to demonstrate 1:1 correspondence and identify how many there are with 100% accuracy on 4 out of 5 trials measured quarterly.

Goal: Given 10 addition problems, Student will independently add single digit numbers with regrouping with 100% accuracy on 4 out of 5 trials as measured quarterly.

Goal:  Student will independently add a single digit number to a double digit number with and without regrouping with 100% accuracy on 4 out of 5 trials measured quarterly.

Goal: Student will independently add double digit numbers to double digit numbers with (or without) regrouping with 100% accuracy on 4 out of 5 trials measured quarterly.

Adding with Number Line:

Goal: Given 10 addition problems and using a number line, Student will independently add single digit numbers with 100% accuracy on 4 out of 5 trials measured quarterly. 

Subtraction:

Goal: Student will independently subtract a single digit number form a double digit number with and without regrouping with 100% accuracy on 4 out of 5 trials measured quarterly.

Goal: Given 10 subtraction problems, Student will independently subtract double digit numbers from double digit numbers with and without regrouping with 100% accuracy on 4 out of 5 trials measured quarterly.

Goal: Student will independently subtract money/price amounts from one another with and without regrouping, while carrying the decimal point with 100% accuracy on 4 out of 5 trials as measured quarterly.

Goal: Using a number line, Student will independently subtract numbers (20 or less) with 100% accuracy on 4 out of 5 trials measured quarterly.

Telling Time:

Goal: Student will independently tell time to the half hour on an analog clock (verbally or written) with 100% accuracy on 4 out of 5 trials measured quarterly. 

Goal: Student will independently tell time to the hour on an analog clock (verbally or written) with 100% accuracy on 4 out of 5 trials measured quarterly.

Elapsed Time:

Goal: Given a problem with a start time and end time, Student will independently determine how much time has elapsed with 100% accuracy on 4 out of 5 trials measured quarterly.

Goal: Given a problem with a start time and duration of activity/event, Student will independently determine what the end time is with 100% accuracy on 4 out of 5 trials measured quarterly.

Dollar More:

Goal: Using the dollar more strategy, Student will independently identify the next dollar up when given a price amount with 100% accuracy on 4 out of 5 trials measured quarterly.

Goal: Student will independently identify the next dollar amount when given a price, determine how much is needed to make the purchase, and count out the necessary amount (using fake school money) with 100% accuracy on 4 out of 5 trials measured quarterly.

Goal: When given a price, student will identify which number is the dollar amount with 100% accuracy on 4 out of 5 trials measured quarterly.      

Money Identification/Counting Money:

Goal: When given a quarter, dime, nickel, and penny, Student will identify the coin and corresponding value with 100% accuracy on 4 out of 5 trials measured quarterly.

Goal: When given a random amount of coins (all of one type), Student will independently count the coins with 100% accuracy on 4 out of 5 trials measured quarterly.

Goal: When given a mix of coins (to include quarter, dime, nickel, penny), Student will independently count the coins with 100% accuracy on 4 out of 5 trials measured quarterly.

Goal: When given a mixture of coins and dollar bills, Student will independently count the money with 100% accuracy on 4 out of 5 trials measured quarterly.

Goal: When give 2, 3, and 4 digit numbers, Student will independently round to the nearest tens, hundreds, thousands independently with 100% accuracy on 4 out of 5 trials measured quarterly.

Greater than/Less than:

Goal: Given 2 numbers, pictures, or groups of items, Student will independently determine which number is greater than/less than/equal by selecting or drawing the appropriate symbol (<,>, =) with 100% accuracy on 4 out of 5 trials measured quarterly.

Goal: Student will independently count objects or pictures of objects and tally the corresponding amount (up to 15) with 100% accuracy on 4 out of 5 trials as measured quarterly.

Goal: Given a number, up to 20, Student will independently tally the corresponding number with 100% accuracy on 4 out of 5 trials measured quarterly.

Goal: Given data and a bar graph template, Student will independently construct a bar graph to display the data and answer 3 questions about the data with 100% accuracy on 4 out of 5 trials measured quarterly.

Goal: Given a line, pie, or bar graph, Student will independently answer questions about each set of data with 100% accuracy on 4 out of 5 trials measured quarterly.

Goal: Given data and a blank graph template, Student will independently construct the graph to display the appropriate data with 100% accuracy on 4 out of 5 trials measured quarterly.

Goal: Student will independently identify the numerator and denominator in a fraction with 100% accuracy on 4 out of 5 trials measured quarterly.

Goal: When given a picture of a shape divided into parts, Student will independently color the correct sections in to represent the fraction given with 100% accuracy on 4 out of 5 trials measured quarterly.

Goal: Student will independently add fractions with like denominators with 100% accuracy on 4 out of 5 trials measured quarterly.

Word Problems:

Goal: Student will independently solve one step addition and subtraction word problems with 100% accuracy on 4 out of 5 trials measured quarterly.

Goal: Student will independently solve two step word problems (mixed addition and subtraction) with 100% accuracy on 4 out of 5 trials measured quarterly.

Goal: Student will independently solve one and two step multiplication world problems with 100% accuracy on 4 out of 5 trials measured quarterly.

Goal: Student will independently read a one or two step word problem, identify which operation is to be used, and solve it with 100% accuracy on 4 out of 5 trials measured quarterly.

Goal: Given a word problem, Student will independently determine which operation is to be used (+,-,x, /) with 100% accuracy on 4 out of 5 trials measured quarterly.

Even/Odd Numbers:

Goal: When given a number, student will independently identify if the number is odd or even (written or verbally), with 100% accuracy on 4 out of 5 trials measured quarterly.

Measurement:

Goal: Given varying lines and objects, Student will independently estimate the length of the object/picture, measure it using a ruler, and identify how long the object/picture is with 100% accuracy on 4 out of 5 trials measured quarterly.

Multiplication:

Goal: Student will independently solve 10 multiplication facts (2, 3, and 5 facts) with 100% accuracy on 4 out of 5 trials measured quarterly.

Goal: Student will independently solve 20 multiplication facts (facts up to 9) with 100% accuracy on 4 out of 5 trials measured quarterly.

Goal: Given a division problem (where the divisor is _____), Student will independently solve it with 100% accuracy on 4 out of 5 trials measured quarterly.

Feel free to use and edit as necessary. It’s up to you how often you want to measure the goals, but remind parents that even if the goal says 5/5 times quarterly, it doesn’t mean you’re only working on it those 5 times. That is just the number of times you’ll take official data. Just make sure it’s a reasonable ## so you have time to take all the data you need. Especially if you have multiple goals/objectives to take data for!

Happy drafting!

Where should we send your

Free lesson plans, success check your email for your free lessons.

We hate SPAM and promise to keep your email address safe.

Want some lesson plans you can immediately use?

Enter your email address below to get 5 free lesson plans now  .

13 SMART Goals Examples for Math Teachers

Math teachers play a role in shaping the minds of young students and fostering a passion for numbers. However being an educator involves continuous learning, self-assessment and setting goals.

Goals serve as our beacon on the path to progress and success. They provide direction, concentrate our efforts , and motivate us to step outside our comfort zones.

For teachers establishing goals is not about enhancing their teaching skills but also about creating an engaging learning environment that promotes positive student outcomes.

This article explores 13 examples of SMART goals that can be integrated into your teaching approach . These objectives are designed to help you refine your strategies boost student involvement and improve math performance.

Whether you’re new to teaching and eager to establish yourself or an experienced educator looking for ideas these targets are tailored to reignite your passion for teaching and propel you toward greater educational achievements.

Table of Contents

What is a SMART Goal?

The SMART approach is a valuable tool for math teachers aiming to enhance their teaching methods and student outcomes. SMART (specific, measurable, attainable, relevant, and time-based) provides a structured framework for setting goals.

• Specific : Set precise goals like improving students’ understanding of algebraic equations.
• Measurable : Track progress with quantifiable targets, such as increasing the class average score in algebra by 20%.
• Attainable : Ensure goals are achievable, considering resources and time.
• Relevant : Align goals with broader educational objectives to foster motivation and perseverance.
• Time-based : Establish clear deadlines for higher student achievement.

Why Every Math Teacher Should Set SMART Goals

SMART goals allow math teachers to enhance teaching effectiveness and promote student success. Setting specific targets helps teachers clarify teaching priorities and tailor instructional strategies to meet diverse student needs.

Measurable goals enable teachers to track progress quantitatively, assess student performance, and identify areas for improvement with clarity and precision.

SMART goals ensure that teachers set achievable and relevant targets, aligning efforts with broader educational objectives and fostering a culture of continuous improvement in the classroom.

By establishing time-bound goals, teachers create a sense of urgency and focus, driving motivation and accountability among students and educators.

Types of SMART Goals for Math Teachers

Below are different forms of SMART goals for math teachers:

Curriculum Goals

Math teachers can establish SMART goals to ensure alignment between curriculum standards and instructional practices. By mapping out learning objectives and assessment strategies, teachers aim to deliver coherent and comprehensive math instruction that meets the needs of diverse learners.

Differentiation Goals

SMART goals here focus on implementing differentiated instruction to address varying levels of student readiness, interests, and learning styles. Teachers may set targets for creating tiered assignments, providing scaffolded support, and integrating technology to personalize learning experiences for each student.

Formative Assessment Goals

Math teachers can develop goals to enhance formative assessment practices, aiming to gather real-time feedback on student understanding and adjust instruction accordingly.

By incorporating strategies such as exit tickets, quizzes, and peer assessments, teachers ensure ongoing assessment that informs instructional decisions and promotes student growth.

Problem-Solving Goals

SMART goals related to problem-solving emphasize the development of students’ mathematical reasoning skills. Teachers may set targets for designing challenging tasks, facilitating mathematical discussions, and encouraging students to apply mathematical concepts in real-world contexts.

Collaboration Goals

The goals here focus on fostering collaboration among math teachers within a school. Teachers may aim to participate in professional learning communities, engage in peer observations and feedback, and collaborate on curriculum development to enhance collective expertise and improve student outcomes.

Technology Integration Goals

These goals involve integrating instructional technology tools and resources to enhance learning experiences. Set targets for using digital platforms, interactive simulations, and multimedia resources to support conceptual understanding and engage students in meaningful math experiences.

13 SMART Goals for Math Teachers

Below are some great examples of SMART goals for math teachers:

1. Boost Math Performance Scores

SMART Goal: “I’ll increase students’ math performance scores by 5% within 6 months. To accomplish this, I want to create specialized assignments and activities that will help to hone math skills and increase math test scores.”

Specific: This outlines what you must do (create specialized assignments and activities) to reach a goal (increasing students’ math performance scores by 5%).

Measurable: You could track the percentage increase in test scores every month.

Attainable: Increasing math performance scores by 5% within 6 months is a realistic goal.

Relevant: This SMART statement relates to your role as a math teacher.

Time-based: You will strive for goal completion over the 6 months ahead.

2. Foster Student Engagement

“To improve student engagement with math, I will develop innovative lesson plans that engage all students regardless of their ability level. By the end of the school year, I want to have students who are more interested in math and willing to participate in class.”

S: The goal is explicit in terms of what needs to be accomplished and the desired outcome.

M: You could measure student engagement by looking at grades, class participation, and survey feedback.

A: This goal is achievable because it involves coming up with creative teaching methods.

R: This statement applies to improving student engagement with math.

T: You have a timeline of one school year to accomplish success.

3. Develop and Expand Math Clubs

“I will work to establish and promote math clubs in the school so that more students can participate in meaningful mathematics-related activities. I plan to develop and implement a system for these clubs by the end of two months.”

S: You’ll work to establish and promote math clubs in the school for two months.

M: Quantify the number of clubs you set up and how many students participate.

A: This is an achievable goal depending on the amount of resources available.

R: The goal is relevant to the primary objective of expanding math clubs in the school.

T: Expect to reach this SMART goal after two whole months.

4. Create Math Resources for At-Home Learning

“I’ll create a library of online math resources, such as websites and videos, to help parents support their students with at-home math instruction within 7 months. I will launch the library within two months and gather feedback for continual improvement.”

S: The math teacher will create a library of online math resources for at-home instruction.

M: You will launch the library within two months and gather feedback.

A: This statement is feasible because the individual has a specific timeline.

R: Creating math resources for at-home instruction is crucial for math teachers.

T: Seven months are required to meet this particular goal.

5. Promote Collaborative Learning

“I will create one collaborative learning project to foster positive relationships between students and promote a sense of community in the classroom. Within a quarter, I plan to observe how the project works and use student feedback to evaluate its effectiveness.”

S: The goal is clearly stated, outlining the objectives and how to gauge success.

M: Through observation of the project’s progress and student feedback, the teacher can evaluate its effectiveness.

A: Creating a collaborative learning project is a doable goal that the math teacher can meet in a quarter.

R: This pertains to student relationships and fostering community in the classroom.

T: You have a quarter (three months) to reach success and observe the results.

6. Build Student Confidence in Math

“I will create an interactive, student-centered environment that encourages and supports students in their learning of math over the next three months. I want to give my students the confidence they need to succeed in math.”

S: This specifies that an interactive environment must be created and last for three months.

M: You could track how frequently students are engaged in the interactive environment.

A: Creating an interactive learning environment within three months is feasible.

R: Math teachers should strive to build their students’ confidence in the subject.

T: Goal achievement is anticipated within a three-month period.

7. Integrate Technology Into Math Lessons

“I want to ensure my students are engaged in math and understand the concepts better, so I’ll experiment with different technologies like video tutorials or interactive online tools to integrate into my math lessons after this school year.”

S: You want to experiment with video tutorials and other technologies for one school year.

M: Monitor how your students’ engagement and understanding of math concepts changes.

A: Using online tools is often easier than many people expect.

R: Integrating technology into math lessons is a great way to keep students engaged.

T: This SMART goal should be met by the end of the school year.

8. Encourage Effective Critical Thinking

“In two months, I’ll create lesson plans that effectively challenge my students’ critical thinking abilities and equip them with the tools to research and analyze information. I expect them to use critical thinking to solve math problems.”

S: Create lesson plans that challenge students’ problem-solving skills and equip them with tools to analyze information.

M: Evaluate success by having students make conclusions in math.

A: This is attainable as you actively take steps to ensure your students learn critical thinking skills .

R: The goal is appropriate because it focuses on developing their student’s critical thinking.

T: This is time-bound because it has an end date of two months.

9. Help Students With Weak Math Skills

“I will create a special program to help students struggling with math within 5 months. The program will be designed to address their weaknesses and push them to succeed. I’ll use a combination of individual instruction, group activities, and practice exams to help improve their math skills.”

S: The goal details the objective, what will be done to achieve it, and the timeline.

M: You could track students’ progress in the program to see how their math skills have improved.

A: This statement is possible within 5 months, given available resources and support.

R: This is important for helping students with weak math skills to succeed in their studies.

T: You should complete the SMART goal after 5 whole months.

10. Improve Instructional Design Skills

“I will take two courses in instructional design and become proficient in creating effective learning experiences within four months. I hope to learn about various instructional design techniques and apply the best strategies for teaching math in my classroom.”

S: This goal states taking courses and learning various instructional design techniques .

M: You can check the number of classes attended, the skills learned, and the strategies you’ve applied in your classroom.

A: Training courses are available online and in person, and you can practice your skills in the classroom.

R: Having instructional design skills is vital for creating a positive learning environment.

T: You have four months to become proficient in the skill.

11. Conduct Regular Math Assessments

“I’ll implement a system of math assessments to identify students’ strengths and weaknesses in the subject. I will complete these assessments quarterly, with the results helping inform my teaching decisions and providing students timely feedback.”

S: This is explicit because it requires you to implement a system of regular math assessments.

M: The teacher can measure through quarterly assessments and student feedback.

A: This SMART goal is achievable with proper preparation and organization.

R: Regular assessments are necessary to identify students’ strengths and weaknesses in math.

T: Goal attainment is expected on a quarterly basis.

12. Use Math Games to Boost Student Interest

“I want to increase student engagement and interest in math by introducing an interactive, game-based curriculum. I plan to develop at least 5 math-based games to use in the classroom by the end of this school year.”

S: The goal is clear. By introducing a game-based curriculum, you want to increase student engagement and interest in math.

M: The math teacher will develop at least 5 math-based games to use in the classroom.

A: Developing 5 math-based games is doable if given the necessary resources and time.

R: This is appropriate for your desire to boost student engagement and interest in math.

T: Long-term success will be met by the end of this school year.

13. Practice Self-Reflection

“I aim to practice self-reflection to gain insight into my teaching methods and learn to become a better educator. I will take 10 minutes daily to write down any successes, failures, or lessons from the day and use this information to identify areas for improvement.”

S: The overall aim is to free up dedicated time to reflect and learn from experiences.

M: This is measured by taking 10 minutes daily for personal reflection.

A: Taking a small amount of time out of the day to reflect is manageable.

R: Personal reflection can lead to insights that help teachers improve their practice.

T: Consider this an ongoing goal, but remember to reflect daily.

FAQs for Math Teachers

How can i tailor smart goals to my math classroom’s specific requirements.

Tailoring SMART goals to your math classroom involves identifying specific needs and objectives. Evaluate student proficiency levels and curriculum requirements. Create goals that directly address these areas, ensuring they are feasible within your classroom context.

How can I collaborate with colleagues or administrators to align these goals with broader educational objectives?

Collaborate with colleagues and administrators to align SMART goals with broader educational objectives. Discuss goals with fellow math teachers to gain insights and share best practices. Seek support from administrators to ensure goals align with school priorities and initiatives.

What role do student assessments play in tracking progress toward SMART goals?

Use a variety of assessment methods to track progress toward SMART goals, such as quizzes, tests, projects, and observations. Analyze assessment data regularly to identify trends and areas for improvement.

How do I ensure SMART goals remain relevant and impactful over time?

Regularly review and adjust SMART goals to maintain their relevance and impact over time. Schedule check-ins to assess progress and evaluate the effectiveness of strategies. Seek feedback from students, colleagues, and administrators to ensure continued effectiveness.

High Impact Tutoring Built By Math Experts

Personalized standards-aligned one-on-one math tutoring for schools and districts

Free ready-to-use math resources

Hundreds of free math resources created by experienced math teachers to save time, build engagement and accelerate growth

20 Effective Math Strategies To Approach Problem-Solving 

Katie Keeton

Math strategies for problem-solving help students use a range of approaches to solve many different types of problems. It involves identifying the problem and carrying out a plan of action to find the answer to mathematical problems.  

Problem-solving skills are essential to math in the general classroom and real-life. They require logical reasoning and critical thinking skills. Students must be equipped with strategies to help them find solutions to problems.

This article explores mathematical problem solving strategies, logical reasoning and critical thinking skills to help learners with solving math word problems independently in real-life situations. 

What are problem-solving strategies?

Problem-solving strategies in math are methods students can use to figure out solutions to math problems. Some problem-solving strategies: 

• Draw a model
• Use different approaches
• Check the inverse to make sure the answer is correct

Students need to have a toolkit of math problem-solving strategies at their disposal to provide different ways to approach math problems. This makes it easier to find solutions and understand math better. 

Strategies can help guide students to the solution when it is difficult ot know when to start.

The ultimate guide to problem solving techniques

Download these ready-to-go problem solving techniques that every student should know. Includes printable tasks for students including challenges, short explanations for teachers with questioning prompts.

20 Math Strategies For Problem-Solving

Different problem-solving math strategies are required for different parts of the problem. It is unlikely that students will use the same strategy to understand and solve the problem. 

Here are 20 strategies to help students develop their problem-solving skills. 

Strategies to understand the problem

Strategies that help students understand the problem before solving it helps ensure they understand: 

• The context
• What the key information is
• How to form a plan to solve it

Following these steps leads students to the correct solution and makes the math word problem easier .

Here are five strategies to help students understand the content of the problem and identify key information. 

1. Read the problem aloud

Read a word problem aloud to help understand it. Hearing the words engages auditory processing. This can make it easier to process and comprehend the context of the situation.

2. Highlight keywords 

When keywords are highlighted in a word problem, it helps the student focus on the essential information needed to solve it. Some important keywords help determine which operation is needed.  For example, if the word problem asks how many are left, the problem likely requires subtraction.  Ensure students highlight the keywords carefully and do not highlight every number or keyword. There is likely irrelevant information in the word problem.

3. Summarize the information

Read the problem aloud, highlight the key information and then summarize the information. Students can do this in their heads or write down a quick summary.  Summaries should include only the important information and be in simple terms that help contextualize the problem.

4. Determine the unknown

A common problem that students have when solving a word problem is misunderstanding what they are solving. Determine what the unknown information is before finding the answer.  Often, a word problem contains a question where you can find the unknown information you need to solve. For example, in the question ‘How many apples are left?’ students need to find the number of apples left over.

5. Make a plan

Once students understand the context of the word problem, have dentified the important information and determined the unknown, they can make a plan to solve it.  The plan will depend on the type of problem. Some problems involve more than one step to solve them as some require more than one answer.  Encourage students to make a list of each step they need to take to solve the problem before getting started.

Strategies for solving the problem 

1. draw a model or diagram.

Students may find it useful to draw a model, picture, diagram, or other visual aid to help with the problem solving process.  It can help to visualize the problem to understand the relationships between the numbers in the problem. In turn, this helps students see the solution.

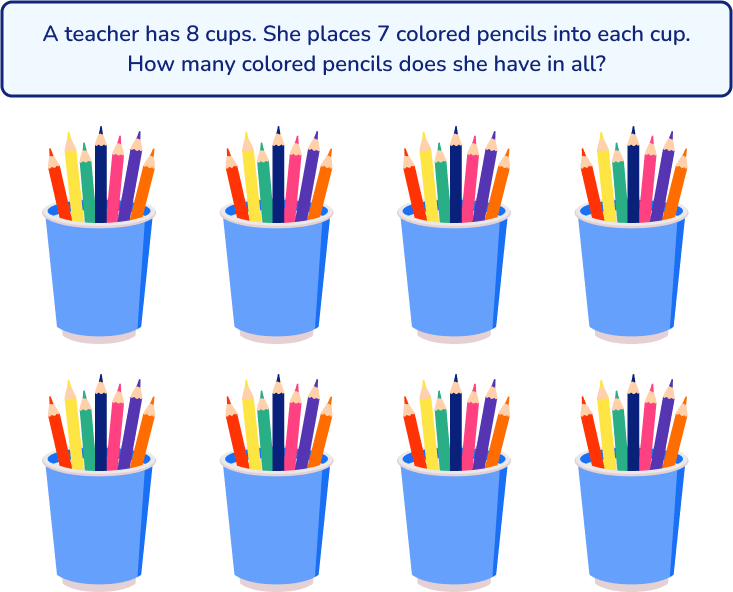
Similarly, you could draw a model to represent the objects in the problem:

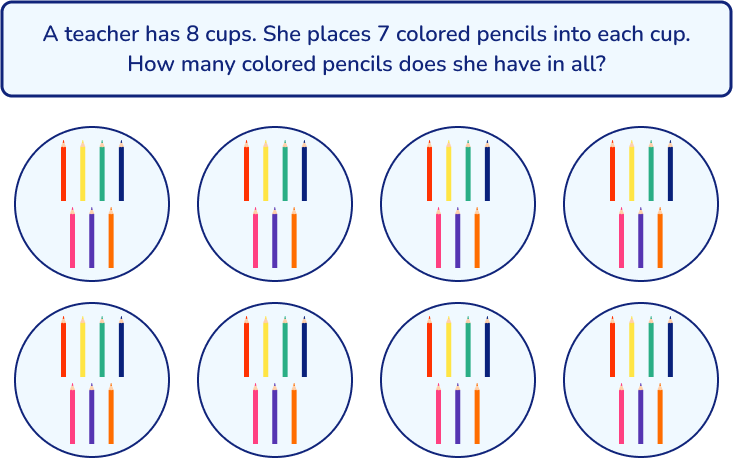
2. Act it out

This particular strategy is applicable at any grade level but is especially helpful in math investigation in elementary school . It involves a physical demonstration or students acting out the problem using movements, concrete resources and math manipulatives .  When students act out a problem, they can visualize and contectualize the word problem in another way and secure an understanding of the math concepts.  The examples below show how 1st-grade students could “act out” an addition and subtraction problem:

3. Work backwards

Working backwards is a popular problem-solving strategy. It involves starting with a possible solution and deciding what steps to take to arrive at that solution.  This strategy can be particularly helpful when students solve math word problems involving multiple steps. They can start at the end and think carefully about each step taken as opposed to jumping to the end of the problem and missing steps in between.

For example,

To solve this problem working backwards, start with the final condition, which is Sam’s grandmother’s age (71) and work backwards to find Sam’s age. Subtract 20 from the grandmother’s age, which is 71.  Then, divide the result by 3 to get Sam’s age. 71 – 20 = 51 51 ÷ 3 = 17 Sam is 17 years old.

4. Write a number sentence

When faced with a word problem, encourage students to write a number sentence based on the information. This helps translate the information in the word problem into a math equation or expression, which is more easily solved.  It is important to fully understand the context of the word problem and what students need to solve before writing an equation to represent it.

5. Use a formula

Specific formulas help solve many math problems. For example, if a problem asks students to find the area of a rug, they would use the area formula (area = length × width) to solve.   Make sure students know the important mathematical formulas they will need in tests and real-life. It can help to display these around the classroom or, for those who need more support, on students’ desks.

Strategies for checking the solution 

Once the problem is solved using an appropriate strategy, it is equally important to check the solution to ensure it is correct and makes sense. 

There are many strategies to check the solution. The strategy for a specific problem is dependent on the problem type and math content involved.

Here are five strategies to help students check their solutions. 

1. Use the Inverse Operation

For simpler problems, a quick and easy problem solving strategy is to use the inverse operation. For example, if the operation to solve a word problem is 56 ÷ 8 = 7 students can check the answer is correct by multiplying 8 × 7. As good practice, encourage students to use the inverse operation routinely to check their work. 

2. Estimate to check for reasonableness

Once students reach an answer, they can use estimation or rounding to see if the answer is reasonable.  Round each number in the equation to a number that’s close and easy to work with, usually a multiple of ten.  For example, if the question was 216 ÷ 18 and the quotient was 12, students might round 216 to 200 and round 18 to 20. Then use mental math to solve 200 ÷ 20, which is 10.  When the estimate is clear the two numbers are close. This means your answer is reasonable. 

3. Plug-In Method

This method is particularly useful for algebraic equations. Specifically when working with variables.  To use the plug-in method, students solve the problem as asked and arrive at an answer. They can then plug the answer into the original equation to see if it works. If it does, the answer is correct.

If students use the equation 20m+80=300 to solve this problem and find that m = 11, they can plug that value back into the equation to see if it is correct. 20m + 80 = 300 20 (11) + 80 = 300 220 + 80 = 300 300 = 300 ✓

4. Peer Review

Peer review is a great tool to use at any grade level as it promotes critical thinking and collaboration between students. The reviewers can look at the problem from a different view as they check to see if the problem was solved correctly.   Problem solvers receive immediate feedback and the opportunity to discuss their thinking with their peers. This strategy is effective with mixed-ability partners or similar-ability partners. In mixed-ability groups, the partner with stronger skills provides guidance and support to the partner with weaker skills, while reinforcing their own understanding of the content and communication skills.  If partners have comparable ability levels and problem-solving skills, they may find that they approach problems differently or have unique insights to offer each other about the problem-solving process.

5. Use a Calculator

A calculator can be introduced at any grade level but may be best for older students who already have a foundational understanding of basic math operations. Provide students with a calculator to allow them to check their solutions independently, accurately, and quickly. Since calculators are so readily available on smartphones and tablets, they allow students to develop practical skills that apply to real-world situations.  

Step-by-step problem-solving processes for your classroom

In his book, How to Solve It , published in 1945, mathematician George Polya introduced a 4-step process to solve problems. 

Polya’s 4 steps include:

• Understand the problem
• Devise a plan
• Carry out the plan

Today, in the style of George Polya, many problem-solving strategies use various acronyms and steps to help students recall. 

Many teachers create posters and anchor charts of their chosen process to display in their classrooms. They can be implemented in any elementary, middle school or high school classroom. 

Here are 5 problem-solving strategies to introduce to students and use in the classroom.

How Third Space Learning improves problem-solving 

Resources .

Third Space Learning offers a free resource library is filled with hundreds of high-quality resources. A team of experienced math experts carefully created each resource to develop students mental arithmetic, problem solving and critical thinking. 

Explore the range of problem solving resources for 2nd to 8th grade students. 

One-on-one tutoring 

Third Space Learning offers one-on-one math tutoring to help students improve their math skills. Highly qualified tutors deliver high-quality lessons aligned to state standards. 

Former teachers and math experts write all of Third Space Learning’s tutoring lessons. Expertly designed lessons follow a “my turn, follow me, your turn” pedagogy to help students move from guided instruction and problem-solving to independent practice. 

Throughout each lesson, tutors ask higher-level thinking questions to promote critical thinking and ensure students are developing a deep understanding of the content and problem-solving skills.

Problem-solving

Educators can use many different strategies to teach problem-solving and help students develop and carry out a plan when solving math problems. Incorporate these math strategies into any math program and use them with a variety of math concepts, from whole numbers and fractions to algebra. 

Teaching students how to choose and implement problem-solving strategies helps them develop mathematical reasoning skills and critical thinking they can apply to real-life problem-solving.

READ MORE :

• 8 Common Core math examples
• Tier 3 Interventions: A School Leaders Guide
• Tier 2 Interventions: A School Leaders Guide
• Tier 1 Interventions: A School Leaders Guide

There are many different strategies for problem-solving; Here are 5 problem-solving strategies: • draw a model  • act it out  • work backwards  • write a number sentence • use a formula

Here are 10 strategies for problem-solving: • Read the problem aloud • Highlight keywords • Summarize the information • Determine the unknown • Make a plan • Draw a model  • Act it out  • Work backwards  • Write a number sentence • Use a formula

1. Understand the problem 2. Devise a plan 3. Carry out the plan 4. Look back

Some strategies you can use to solve challenging math problems are: breaking the problem into smaller parts, using diagrams or models, applying logical reasoning, and trying different approaches.

Related articles

Differentiation Strategies In The Classroom: 8 Methods For Every Teacher

Retrieval Practice: A Foolproof Method To Improve Student Retention and Recall

5 Tried And Tested Strategies To Increase Student Engagement At School

Rote Memorization: Is It Effective In Education?

Ultimate Guide to Metacognition [FREE]

Looking for a summary on metacognition in relation to math teaching and learning?

Check out this guide featuring practical examples, tips and strategies to successfully embed metacognition across your school to accelerate math growth.

Privacy Overview

• Big Ideas Login
• Request a Sample

Big Ideas Learning’s Mathematics Teaching Practices Series: Implementing Tasks That Promote Reasoning and Problem Solving

• Sophie Murphy
• August 25 2020

In this series, we will explore the eight research-based essential Mathematics Teaching Practices found in NCTM’s Principles to Actions. Today’s blog focuses on the second essential Mathematics Teaching Practices: implementing tasks that promote reasoning and problem-solving.

Today’s blog focuses on the second of NCTM’s eight research-based essential Mathematics Teaching Practices: implementing tasks that promote reasoning and problem solving.

Mathematics Teaching Practice #2 - Implementing Tasks That Promote Reasoning and Problem Solving

The second Mathematics Teaching Practice is an essential stepping-stone for understanding and transferring mathematical concepts and understanding. For students to successfully engage in deep-level tasks that allow for reasoning and problem solving, their mathematics classrooms cannot limit their thinking to pure memorization and carrying out computations with little or no understanding. Students need to explore and investigate mathematical reasoning with confidence and understanding.

In order to implement tasks that promote reasoning and problem solving, teachers and students must aim to do the following.

Teachers must:

• Provide opportunities for students to engage in deep-level learning.
• Motivate students’ learning of mathematics through opportunities for exploring and solving problems that build on and extend their current mathematical understanding.
• Choose and develop tasks that provide multiple entry points for problems to be solved.
• Pose tasks on a regular basis that require a high level of cognitive demand.
• Know their students to find the “Goldilocks Zone” – not too easy, not too hard (or boring!)
• Support students in exploring tasks that connect to real-world mathematics.
• Encourage students to use procedures and strategies to make connections which can then be applied to solving tasks.
• Value procedural and conceptual thinking. The goal is flexible and transferable thinking from one concept to another.

Students must:

• Understand that learning can be difficult but embrace the challenge by persevering in solving problems.
• Move into deep-level learning at the right time (after moving through sufficient surface level) to reason through tasks.
• Use goals to understand where they are, where they need to go, and how they are going to get there through goal setting and clarity of task/understanding.
• Draw upon and make connections with prior understanding and ideas when provided with challenging and deeper-level tasks.
• Use tools and representations as needed to support thinking and problem solving.
• Use a variety of solution approaches to solve problems.
• Move from surface understandings to deeper understandings by defining, describing, discussing, and justifying to one another.
• Work to make sense out of tasks and persevere in solving problems, and if the problems are too challenging, be able to return to the surface-level knowledge to gain further understanding and skills in solving the tasks.

Research Behind Deep-Level Learning - Reasoning and Problem Solving in Mathematics

Deep learning is hard. It requires a cognitive demand that can be challenging. It often requires prior learning and the ability to compare and contrast, apply previous knowledge, and make connections. Unless the right conditions are in place to learn how to reason, many students are left at the surface and procedural level of mathematical understanding that relies primarily on using memory alone. Or, on the flip side, students are not ready to go deep, as they have limited skills and or knowledge to move into deeper levels of understanding, reasoning, and problem solving.

If students get into deep-level learning too quickly, they can lose confidence and self-efficacy about their mathematical ability. For this reason, we need to support all students to become engaged in high-level thinking and ensure that all students have the opportunity to experience reasoning and problem solving throughout a sequence of learning, not just those who are considered to be high achievers in mathematics.

When multiple procedures are required, there is a need to understand the surface level, yet find the right balance to move from surface to deep so that students can solve and understand more complex mathematical problems. Hattie (2012) defines this as the “Goldilocks Zone,” where the learning is not too easy and not too hard (or tedious) – it is just right for all learners!

Stein and Lane (1996) suggest that student learning has the greatest impact in classrooms where the learning tasks consistently encourage high-level student thinking and reasoning and the least impact in classrooms where the tasks are routinely procedural in nature. It is well documented that deeper-level mathematical tasks are often perceived to be more complex and difficult to deliver in the classroom and that tasks that are procedural with fewer cognitive demands are prioritized in place of deeper-level instruction (Boaler, 1997).

The Importance of Using a Taxonomy

A taxonomy that is used effectively can encourage reasoning and access to the mathematics through multiple entry points that have supported students through the important learning and understandings being developed prior to deeper-level learning. This includes the use of different representations and tools that foster problem solving through a variety of solution strategies that move through different levels of learning. Furthermore, teachers can use a learning taxonomy to support the appropriate use and development of discourse and thinking.  

A taxonomy can be used to support success criteria that moves from surface to deep level as the sequence of learning progresses. For example, using ‘I can,’ success criteria statements move students through the learning as the cognition progresses.

When effectively using a taxonomy, mathematical tasks are viewed as placing higher-level cognitive demands on students as they are encouraged to engage in active inquiry and exploration or to use procedures in ways that are meaningfully connected with concepts or understanding. Tasks that encourage students to use procedures, formulas, or algorithms in ways that are not actively linked to meaning, or that consist primarily of memorization or the reproduction of previously memorized facts, are viewed as placing lower-level cognitive demands on students.

A taxonomy should not be perceived as a checklist, but instead as a framework and chance to move from procedural to conceptual understandings. This gives students the opportunity to transfer their understandings from one context to another as teachers move from placing lower-level cognitive demands on students to higher cognitive demands that require reasoning and problem solving.

The SOLO Taxonomy

The Structure of Observed Learning Outcomes (SOLO) Taxonomy was developed by Biggs & Collis in 1982.

The SOLO taxonomy consists of two major categories with increasingly complex stages:

• Surface (uni-structural and multi-structural responses)
• Deep (relational and extended abstract responses).

Within the two categories, the SOLO taxonomy consists of four levels (Biggs & Collis, 1982):

• Multiple ideas
• Relating the ideas
• Extending the ideas

Biggs and Collis argue that the purpose of the Structured Observed Learning Outcome (SOLO) taxonomy is to balance deep knowledge with surface knowledge when preparing students for deep-level understandings and transferring these to new contexts.

Each level of the SOLO taxonomy increases the demand on the amount of working memory or attention span. At the surface levels, a student only needs to encode the given information and can use a recall strategy to provide an answer. At the deep levels, a student needs to think not only about more things at once, but also how those objects interrelate.  

The SOLO taxonomy is used extensively throughout the Big Ideas Math Series to inform the progression of learning and design goals through the consistent implementation of learning targets and success criteria .

How Can Teachers Effectively Promote Reasoning and Problem Solving?

As outlined in this blog and the prior blog, the use of learning intentions and success criteria are vital in providing instructional practice that offers students clear and purposeful goals and a foundation for what success looks like. The learning intentions should plainly explain what students need to understand and what they should be able to do. In addition, this also helps teachers plan learning activities. 

Professor John Hattie’s research demonstrates that having clear  learning intentions and success criteria ​ coupled with the support of a taxonomy/framework that moves the students from procedural understandings to more complex deeper understandings help students self-regulate. When teachers set and communicate clear lesson goals to help students understand the success criteria, students know where they are, where they need to go, and how they are going to get there. They move from the procedural through to the conceptual. They cannot move to conceptual without the surface-level procedural.

You can find effective examples of  learning intentions (also known as learning targets) and success criteria in the Big Ideas Math Series . These align to both the learning within the series and the teacher notes, providing teachers with an excellent starting point for their teaching strategies.

Key Takeaways

When promoting reasoning and problem solving, we suggest that teachers should consider using a taxonomy. Using a framework such as the  SOLO Taxonomy can help teachers to ensure that tasks include lower and higher-level understanding, skills, and knowledge and that students are encouraged to engage in higher-level, problem-solving tasks that build up from lower-level cognitive skills. In order for students to learn mathematics with understanding, they must have opportunities to regularly engage in tasks that allow for reasoning and problem solving and make possible multiple entry points and varied solution strategies.

Remember, deep-level learning is hard, but we embrace challenge. Surface-level understandings are just as important to helping students to reason and problem solve as deep-level understandings.

Together with Big Ideas Learning and National Geographic Learning, I will continue to provide you with support as we navigate the complexities of 2020, whether this is online and remotely or back in the classroom. Despite the challenges, we are committed to supporting teachers in moving forward with high impact teaching and learning, starting with NCTM’s Mathematics Teaching Practices.

We will continue to share practical examples and connections to the NCTM evidence-based Mathematics

Teaching Strategies that will impact all classroom environments when used explicitly and intentionally. 

Keep in touch, ask any questions or comment via twitter ( @_sophie_murphy_ ) and through  Big Ideas Learning  and  National Geographic Learning . Stay safe and well. I look forward to connecting with you all again soon.

Biggs , J., &  Collis , K. ( 1982 ). Evaluating the quality of learning The SOLO taxonomy . New York Academic Press.

Hattie, J. (2012).  Visible learning for teachers: Maximizing impact on learning . Routledge.

Henningsen, M., & Stein, M. K. (1997). Mathematical tasks and student cognition: Classroom-based factors that support and inhibit high-level mathematical thinking and reasoning.  Journal for research in mathematics education , 524-549.

Stein, M. K., & Smith, M. (1993). Practices for orchestrating productive mathematics discussions.  Reston, VA: National Council of Teachers of Mathematics .

Related Articles

Interview with laurie boswell: supporting math educators.

Topics: Resources , Professional Development , Student Engagement

10 Summer Reads for Teachers

Topics: Resources , Professional Development , Educator Empowerment

Big Ideas Learning’s Mathematics Teaching Practices Series: Establishing Mathematics Goals to Focus Learning

Topics: Professional Development

10 Ways to Teach Problem Solving in Math

10 ways to teach problem solving in math: effective strategies and approaches.

Problem-solving is a fundamental skill in mathematics education that goes beyond mere calculation. It encourages students to think critically, analyze situations, and apply mathematical concepts to real-world scenarios. 

In this comprehensive guide, we’ll explore various strategies and approaches for teaching problem-solving in math, helping educators empower their students with essential skills for academic success and beyond.

Table of Contents

Understanding the importance of problem-solving in math.

Problem-solving lies at the heart of mathematics. It involves identifying issues, analyzing information, and developing solutions using mathematical knowledge and skills. Teaching problem-solving in math is crucial because it:

• Develops critical thinking skills
• Enhances logical reasoning abilities
• Improves mathematical comprehension
• Prepares students for real-world challenges
• Boosts confidence in mathematical abilities
• Encourages creativity and innovation
• Promotes perseverance and resilience

By focusing on problem-solving, educators can help students develop a deeper understanding of mathematical concepts and their applications in various contexts.

10 Key Strategies for Teaching Problem-Solving in Math

1. encourage multiple approaches.

One of the most effective ways to teach problem-solving is to encourage students to explore multiple approaches to solving a problem. This strategy helps students understand that there’s often more than one way to arrive at a solution, fostering creativity and flexibility in their thinking.

Implementation steps:

• Present a problem to the class.
• Ask students to brainstorm different ways to approach the problem.
• Have students share their ideas with the class.
• Discuss the pros and cons of each approach.
• Encourage students to try different methods and compare results.

2. Teach Problem-Solving Steps

Introduce students to a structured problem-solving process. A common approach includes these steps:

• Understand the problem : Read carefully and identify key information.
• Devise a plan : Choose an appropriate strategy.
• Carry out the plan : Implement the chosen strategy.
• Look back and reflect : Check the solution and consider alternative approaches.

This framework, often attributed to mathematician George Pólya, provides a systematic approach to tackling mathematical problems.

• Introduce the problem-solving steps to students.
• Model the process using a sample problem.
• Provide guided practice with a new problem.
• Encourage students to use the steps independently.
• Reflect on the effectiveness of the process.

3. Use Visual Representations

Visual aids can be powerful tools in problem-solving. Encourage students to use diagrams, charts, or graphs to represent problems visually. This can help them better understand the problem and identify potential solutions.

• Introduce various types of visual representations (e.g., diagrams, charts, graphs).
• Demonstrate how to create and use visual aids for problem-solving.
• Provide practice problems that lend themselves to visual representation.
• Encourage students to share and explain their visual representations.
• Discuss how visual aids contribute to problem-solving.

4. Incorporate Real-World Problems

Connecting math problems to real-life situations makes problem-solving more relevant and engaging for students. Use examples from everyday life to demonstrate how mathematical concepts apply in practical scenarios.

• Collect real-world problems relevant to students’ lives and interests.
• Present these problems to the class.
• Discuss how mathematical concepts relate to the real-world situations.
• Have students create their own real-world math problems.
• Encourage students to share and solve each other’s problems.

5. Promote Collaborative Learning

Group problem-solving activities can enhance learning by allowing students to share ideas, discuss strategies, and learn from each other. This approach also helps develop communication skills crucial for explaining mathematical thinking.

• Divide the class into small groups.
• Assign challenging problems that require collaboration.
• Encourage group discussions and brainstorming.
• Have groups present their solutions to the class.
• Facilitate a class discussion on different approaches and solutions.

6. Teach Estimation Skills

Estimation is a valuable problem-solving tool. It helps students quickly assess whether their solutions are reasonable and can guide them towards correct answers.

• Introduce the concept of estimation and its importance.
• Teach various estimation strategies (e.g., rounding, benchmarking).
• Provide practice problems for estimation.
• Compare estimated answers with exact calculations.
• Discuss when and how to use estimation in problem-solving.

7. Emphasize Process Over Answer

While correct answers are important, focusing on the problem-solving process helps students develop a deeper understanding of mathematical concepts. Encourage students to explain their thinking and justify their solutions.

• Create a classroom culture that values process over just the final answer.
• Ask students to explain their problem-solving steps.
• Provide opportunities for students to share different approaches.
• Use rubrics that assess both process and final answer.
• Encourage students to reflect on their problem-solving strategies.

8. Use Open-Ended Questions

Open-ended questions encourage creative thinking and allow for multiple correct answers. They challenge students to think beyond routine procedures and explore various problem-solving strategies.

• Introduce the concept of open-ended questions in math.
• Provide examples of open-ended math problems.
• Have students create their open-ended questions.
• Discuss and compare different solutions to open-ended problems.
• Reflect on how open-ended questions promote deeper thinking.

9. Incorporate Technology

Utilize educational technology tools and software to enhance problem-solving instruction. Many interactive platforms offer engaging problem-solving activities that can supplement traditional teaching methods.

• Research and select appropriate math problem-solving software or apps.
• Introduce the chosen technology to students.
• Provide guided practice using the technology.
• Assign technology-based problem-solving activities.
• Discuss how technology can aid in problem-solving.

10. Teach Metacognitive Strategies

Help students develop metacognitive skills by encouraging them to reflect on their problem-solving processes. This self-awareness can lead to more effective learning and problem-solving strategies.

• Introduce the concept of metacognition.
• Model metacognitive thinking during problem-solving.
• Provide prompts for students to reflect on their thinking.
• Have students keep a problem-solving journal.
• Discuss and share metacognitive strategies as a class.

Implementing Problem-Solving in the Math Curriculum

1. regular problem-solving sessions.

Dedicate specific class time to problem-solving activities. This consistent practice helps students develop and refine their skills over time.

• Schedule regular problem-solving sessions (e.g., weekly).
• Select a variety of problems that target different skills and concepts.
• Use a mix of individual, pair, and group problem-solving activities.
• Provide opportunities for students to share and discuss solutions.
• Reflect on problem-solving strategies used in each session.

2. Cross-Curricular Integration

Incorporate problem-solving across different subjects to demonstrate its wide-ranging applications and reinforce its importance beyond math class.

• Collaborate with teachers from other subjects.
• Identify areas where math problem-solving can be applied in other subjects.
• Develop cross-curricular problem-solving activities.
• Have students reflect on how math problem-solving relates to other subjects.
• Showcase cross-curricular problem-solving projects.

3. Differentiated Instruction

Provide problems at various difficulty levels to cater to different student abilities and ensure all students are appropriately challenged.

• Assess students’ current problem-solving abilities.
• Create or select problems at different levels of difficulty.
• Allow students to choose problems that match their skill level.
• Provide scaffolding for struggling students.
• Offer extension activities for advanced problem solvers.

4. Ongoing Assessment

Regularly assess students’ problem-solving skills to track progress and identify areas for improvement. Use a mix of formative and summative assessments.

• Develop rubrics for assessing problem-solving skills.
• Use formative assessments during problem-solving activities.
• Implement periodic summative assessments focused on problem-solving.
• Provide constructive feedback on problem-solving strategies.
• Use assessment data to inform instruction and intervention.

Overcoming Challenges in Teaching Problem-Solving

1. student frustration and anxiety.

Some students may become frustrated when faced with challenging problems. Encourage a growth mindset and provide scaffolded support to build confidence.

Strategies:

• Normalize struggle as part of the learning process.
• Teach specific strategies for managing frustration.
• Provide encouragement and positive reinforcement.
• Break down complex problems into smaller, manageable steps.
• Celebrate effort and progress, not just correct answers.

2. Time Constraints

Problem-solving activities can be time-consuming. Plan lessons carefully and consider incorporating problem-solving into homework assignments.

• Prioritize problem-solving in lesson planning.
• Use a flipped classroom approach for some problem-solving activities.
• Assign problem-solving homework with clear guidelines.
• Implement efficient classroom routines for problem-solving sessions.
• Use technology to extend problem-solving beyond class time.

3. Diverse Learning Needs

Students have varying abilities and learning styles. Use a range of problem types and difficulty levels to accommodate all learners.

• Implement Universal Design for Learning principles.
• Offer problems in multiple formats (visual, auditory, kinesthetic).
• Provide options for how students can demonstrate their problem-solving.
• Use flexible grouping strategies.
• Offer individualized support and challenges as needed.

4. Resistance to Non-Routine Problems

Students accustomed to routine exercises may resist more complex problem-solving tasks. Gradually introduce more challenging problems and emphasize the value of the problem-solving process.

• Start with familiar contexts and gradually increase complexity.
• Explicitly teach the value of tackling non-routine problems.
• Provide structured support for complex problems.
• Encourage students to embrace challenges as learning opportunities.
• Highlight the real-world relevance of non-routine problem-solving.

One-to-One Online Tutoring for Personalized Problem-Solving Help

While classroom instruction is vital, some students require additional support to master problem-solving in math. In such cases, personalized tutoring can make a significant difference.

Online tutoring websites offer personalized one-to-one virtual sessions, helping students who struggle with math concepts or problem-solving. Here are some key benefits of personalized tutoring:

• Tailored Instruction : Tutors design lessons according to the student’s unique strengths and weaknesses, addressing gaps in understanding.
• Step-by-Step Guidance : Tutors walk students through problems, ensuring they fully grasp each concept and solution process.
• Interactive Learning : With live virtual tutoring, students can ask questions and receive real-time feedback, helping them stay engaged and improve their skills.

Platforms like Guru at Home offer affordable, flexible scheduling, making it easy for students to get the help they need when they need it.

Read More – 6 Ways to Enhance Problem-Solving Skills through Personalized Tutoring

Teaching problem-solving in math is essential for developing students’ critical thinking , creativity, and perseverance. By incorporating a variety of strategies, including visual aids, collaborative learning, and real-world applications, educators can foster a classroom environment that promotes effective problem-solving. With regular practice and support, students can become confident problem solvers who are well-prepared for future academic and life challenges.

Teaching problem-solving in math enhances critical thinking, logical reasoning, and mathematical comprehension. It prepares students for real-world challenges and fosters creativity, confidence, and perseverance.

Incorporate real-world problems, encourage multiple approaches, and use visual aids. Group activities and technology-based tools can also make problem-solving more engaging.

Provide structured support, such as breaking problems into smaller steps, offering visual aids, and encouraging collaboration with peers. Emphasizing the problem-solving process over the final answer can also help build confidence.

Use a mix of formative and summative assessments, including problem-solving journals, group activities, and rubrics that evaluate both process and outcome.

Collaborate with other teachers to identify cross-curricular problem-solving opportunities. Develop projects that require applying mathematical problem-solving in subjects like science, economics, or geography.

Related Posts

15 benefits of online tutoring for kids in 2022 – guru at home, how to write a diary for kids, : every student must know.

Our support is available to help you 24 hours a day, seven days a week.

• +918440868293
• +917014835141
• [email protected]
• +91 99451 65900

• +1 251 901 5077

Academics (K12)

Online tutoring subjects, other popular courses, international.

Copyright © 2024 Guru at home – All Rights Reserved.

Get a Free Academic Counselling Session